| Discrete Dynamics Lab |
The
![]() -rule:
universal computation in a non-isotropic Life-like Cellular Automaton
Journal of Cellular Automata, Vol 10. No.3-4, 261-294,
arXiv preprint pdf -- 1.8M
-rule:
universal computation in a non-isotropic Life-like Cellular Automaton
Journal of Cellular Automata, Vol 10. No.3-4, 261-294,
arXiv preprint pdf -- 1.8M
José Manuel Gómez Soto
Universidad Autónoma de Zacatecas,
Unidad Académica de Matemáticas. Zacatecas, Zac. México,
jmgomezuam@gmail.com,
http://matematicas.reduaz.mx/~jmgomez
Andrew Wuensche
Discrete Dynamics Lab, London, UK,
andy@ddlab.org, http://www.ddlab.org
We present a new Life-like cellular automaton capable of logic universality -- the X-rule. The CA is 2D, binary, with a Moore neighborhood and λ parameter similar to the game-of-Life, but is not based on birth/survival and is non-isotropic. We outline the search method. Several glider types and stable structures emerge spontaneously within X-rule dynamics. We construct glider-guns based on periodic oscillations between stable barriers, and interactions to create logical gates.
The site is still under construction, but some details from the paper are shown below:
Gc glider-guns are compound, made by combining Ga glider-guns and eaters,
click to enlarge Gc gliders, period 4, speed=c/2
|  Gc glider-gun North
| 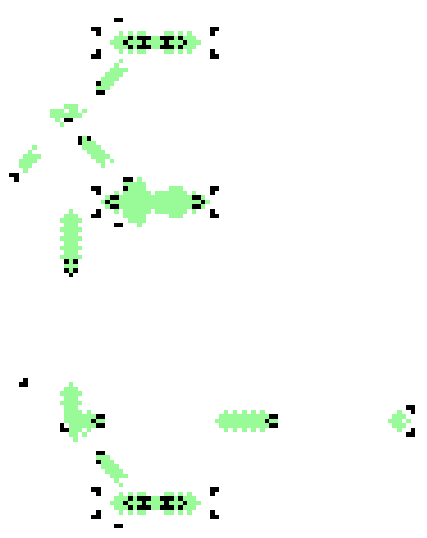 Gc glider-gun South then East
| 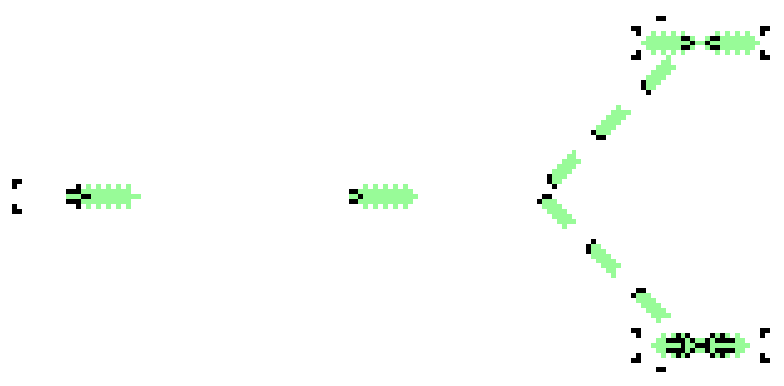 Gc glider-gun West | ||
More compound glider-guns made fron Ga and Gc glider-guns, and eaters
click to enlarge|
|  Gc glider-gun South then Ga NE
|  Gc glider-gun West | |
Logical gates NOT, AND OR and NAND, with output towards the WEST
click to enlarge|
| 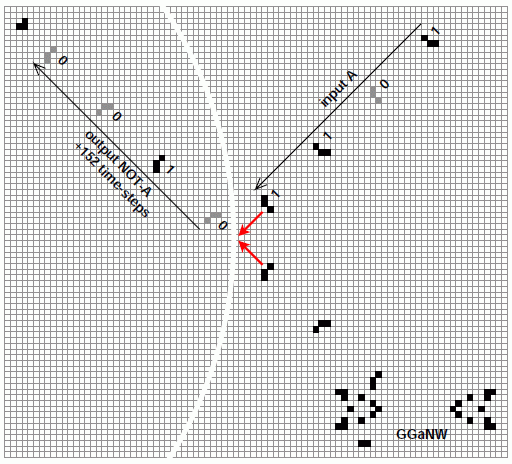 NOT logical gate
| 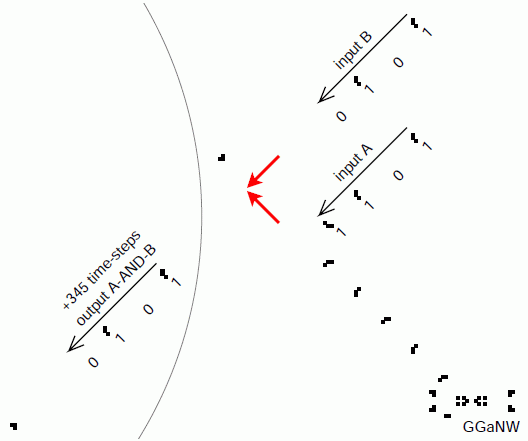 AND logical gate
|  OR logical gate
| 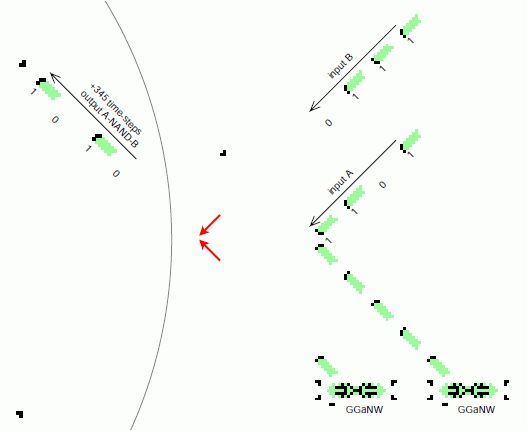 NAND logical gate | |||
Logical gates NOT, AND OR and NAND, with output towards the East
click to enlarge|
| 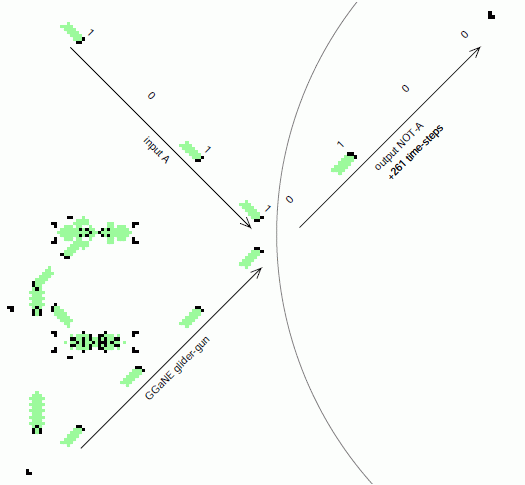 NOT logical gate
|  AND logical gate
|  OR logical gate
| 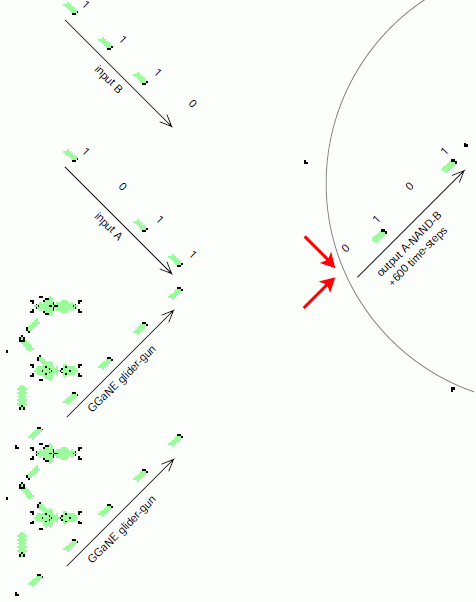 NAND logical gate | |||